Education Archives - The Silent Knight

Will 2020 See The Extinction Of The Red-eyed Monks?

For those of you not familiar with what has been called “the hardest logic puzzle in the world”^Link, The Blue-eyed Monk problem or Blue-eyed Islander problem (Another version), check out the above reference links. For what it’s worth, that “hardest” title is probably an exaggeration. But my new version, inspired by recent events, might be harder.

In this version of the famous logic problem, there are a number of monks on this remote island. All of them are “perfect logicians”. Every monk sees every other monk every day, but they are rugged individualists who never talk to each other or communicate in any way. They also take full responsibility for their actions and obey all rules and protocols. (Yes, this problem is entirely fictional.) Strangely, there are no mirrors or reflective surfaces on the island. The ferry visits the island every night to drop off supplies. It would take the monks to the mainland, if necessary. But none of the islanders wants to leave and no strangers are allowed on the island. In this version of the problem, all of the monks have red eyes.

One day, all but one of the monks noticed that one of their peers had blue eyes. But they all continue to go about their lives. Many days later, almost all of them notice that two of the monks now have blue eyes. Still, life goes on. This trend continues with a slowly increasing frequency until many, many days later, the first monk to have developed blue eyes is found dead. Doctors on the mainland soon discover that some as-yet-unknown, but contagious pathogen hit the island. The number of monks infected has been growing 2% every day for quite some time. The only visible symptom is the changing of eye color from red to blue. The disease is not contagious until the eyes go blue but then remains contagious until the infected person dies. How it spreads is still unknown, but obviously, direct contact or even close proximity is not required.

By the time the doctors figure all of this out, red-eyed monks have started changing eye color at the rate of one every other day and the second blue-eyed monk has just died. If a blue-eyed monk gets to the mainland in time, they can be cured. but the ferry does have a limited capacity. The monks are pacifists, so there will be no shooting of blue-eyed monks (yes, I might have a personal stake in this directive). All of this information was left on a large sign at the ferry dock the next day.

So what do the monks do? Will they go extinct? Will 2020 see the end of all logical thought on this planet? Only you can answer that question. Good luck! Leave your answers in the comment section below.

To see the Note click here.To hide the Note click here.

In the interest of full disclosure and transparency, I have addressed the original monk problem before. After much fumbling, I did come up with a better (faster) solution, which was widely regarded as cheating (see A Better Solution to the Blue-eyed Monk Problem.

Truth In Advertising: An Unborn Baby’s Beating Heart

Above is one version of a common billboard along the interstates in Florida and across America. The problem for me is that the spokesman didn’t look anything like that when he was 18 days from conception. He would have been barely visible and a lot less cuddly. Below, I’ve taken the liberty to replace him with his earlier self, which would have been about half the size of a pea or just able to fit through the pupil in the eye of his older self as shown in the background of the revised billboard. His heart would have been larger than a grain of sand. He still has no eyes, arms, or lungs, and I’ve found no information on brain function. I don’t think he’s feeling any pain. Now ain’t that cute?

His Odds Of Success (Birth)

While I have touched on this subject before (in my article Save Your Birthday – Vote For Clinton), this time I dug deeper in order to put things into a more serious perspective. Here’s what I found:

According to ABORT73.COM, abortions have been decreasing slightly every year for the last decade. 879 thousand occurring in the United States in 2017.
The Statistica website says that the number of births in this country has been fairly stable. There were about 3.86 million of them in 2017.
The attrition rate of fertilized human eggs is not as well known. Comparing articles on spontaneous abortion in humans and Early embryo mortality in natural human reproduction, it looks like only 25% of fertilized eggs actually make it to birth (± at least 10%).

Here’s what that means: if you start with 18 fertilized human eggs, 13 will die from natural causes before birth, and one embryo or fetus would be aborted. Both forms of attrition are “front-loaded”. 2/3 of abortions happen by the 8th week^A1, while 2/3 of fertilized eggs will die in their first 11 weeks^A2.

To see the Note click here.To hide the Note click here.

A common mistake would be to compare the number of abortions with the number of births directly but to compare apples to apples, as they say, you need to use the same reference point. Here’s the math:

3.86 million births divided by 25% (or 0.25) gives 15.4 million fertilized eggs. That would be the common reference point. Divide that by 879 thousand abortions and you get the total number of fertilized eggs there are for every abortion (17.6 – let’s round to 18). To find the number of those fertilized eggs that would die naturally, simply multiply the same 18 by 75% (or 0.75).

One thing that is not clear is “what are the odds that the aborted “child” would have died from natural causes anyway?” The easy answer (and my best guess so far) is 75%. That means there are a lot of self-righteous people out there (many being middle-aged white males with nothing invested) who are willing to throw a woman in jail for long periods of time without even caring about the circumstances of her case to protect something that probably wasn’t going to make it anyway. I guess for the pious, any excuse is a good one for beating up on your neighbor.

At least that’s the way I see it. Any comments?

Has the Republican Party Become a Ponzi or Pyramid Scheme?

To see the Note click here.To hide the Note click here.

Definition of a pyramid scheme.

As a teacher, I didn’t heartily endorse “the Curve.” I didn’t believe that just because a student could find five friends who were either stupider or lazier than they were they deserved a free pass. (I’m sure that for some of my students that was no trivial feat). This also tied into a theory I developed early in my teaching career^Article (which turned into a prophesy). Unlike doctors, who are said to be able to bury their mistakes, if teachers were to make mistakes, and for political or social reasons were unable to correct the system to reduce the number of those mistakes, then non-educated people could eventually reach critical mass. They could then elect enough unqualified politicians to negatively affect the education process (with budget cuts, for example). This would be the beginning of a feedback loop that could put American education and thus American society on a death spiral. But that could never happen here, right?

Now that Mr. Trump has been elected president and his conduct, unchecked by congress, has become more and more outrageous, I find it interesting to watch some of his supporters as they have to warp their world (or the principles by which that world is defined) to greater degrees in order to be able to justify His actions within that small world. To do that successfully, they need other people (their five friends) to be dumb enough to fall for those contorted explanations. As time goes on, those friends need to recruit their own set of even dumber friends so that they don’t look like the fools that they are . . . and so on. But even now, dumb and foolish people are not an unlimited resource in this country. And Trump’s actions will continue to test that resource like no other leader in the history of the Earth. So as in all such schemes, the buffoon bubble is bound to break. The only question is – will that happen before the next election? I’m not so sure it will. Any comments?

Please Help! The Special Theory Of Relativity Is Haunting (or is it Taunting) Me!

A long time ago (when I was in the sixth or seventh grade) in a galaxy far, far away (namely Southern California), I was introduced to Einstein’s Special Theory of Relativity by way of a story about two astronauts on different spacecraft watching a bouncing-light-beam clock, and I was really impressed. But upon further review as I was chewing my cud (See definitions of “ruminate”), as I was wont to do walking home from school, it didn’t seem to make as much sense. I developed some questions but didn’t know where to get answers, and as life pressed on my attention wandered elsewhere, and everyone lived happily ever after. . .

Until recently. In the last year, the subject has come up several times, the questions seem to be the same, and I still don’t know where to turn.

The Story

Although not exactly as I remember it, www.dummies.com describes a similar thought experiment in the second section, “Unifying space and time”, with a spacecraft traveling at ½ the speed of light, but doesn’t give much explanation. The Star Garden gives a more detailed explanation.

The Problem

“Time Dilation”, Section 7.2.2 of Reference 2 concludes

“The time between heartbeats is also slower, and so from the perspective of a stationary person, a moving person appears to be living their life at a slower rate. Conversely, from the perspective of the moving person, the stationary person seems to live their life as if it is being fast-forwarded. If they travel fast enough, then they could see the stationary person age before their eyes.”

My problem with that conclusion is that based on the second paragraph of Section 7.1 at the beginning the article, which states

“there’s no such thing as absolute speed or velocity, and something can only be said to be moving at a constant velocity relative to something else. In the same way, something can only be said to be stationary relative to something else”,

how do we really know which astronaut is supposed to be aging before our eyes? What if we put a bouncing-light clock on each spacecraft? Would it explode?

The authors of Reference 2 seem to address this issue at the bottom of the next section, 7.2.3, where they say

“The twin paradox

The twin paradox asks why the astronaut can consider themselves to be moving and the Earth to be stationary, when Galileo’s relativity shows that there’s no such thing as absolute velocity. Why can’t the astronaut consider themselves to be stationary while the Earth moves away at tremendous speeds?

The answer is acceleration. Galileo’s relativity applies to inertial – that is non-accelerating – reference frames. The fact that the astronaut must have accelerated before getting to such high speed means that they know they are the one that is moving.”

To me, this sounds bogus; any acceleration before or after the experiment should be immaterial. Let’s have three observers; one person remains on Earth while two astronauts board different spacecraft, each leaving the Earth in opposite directions and reaching similar stable speeds well in excess of ½ the speed of light (meaning their relative speed would exceed the speed of light in a non-relativistic world). Each of the observers has their own bouncing-light clock. If you start counting after their speed stabilizes, exactly how do each of the three observers see the ages change for the other two?

One Last Question

A question that one might ask in each of these scenarios is “how does the state of the bouncing light in one spacecraft become known to the other observers?” Reference 1 states that Amber, on a different spacecraft, would see the bouncing light travel further between bounces, as if Amber had super X-ray vision and/or was otherwise experiencing the light beam in real time. How does that work? If she had to wait for reflected light rays from the event to reach her eyes, would that affect the apparent outcome in any way?

One phenomenon that may or may not have anything to do with the solution to this problem involves ocean waves. In deep water, a wave’s speed is nearly proportional to the square root of its wavelength^A,

To see the Note click here.To hide the Note click here.

$S \cong 1.251 \sqrt{W}$

where S is the wave’s speed (measured in meters per second) and W is its wavelength (in meters).
For shallow water waves, the speed is proportional to the square root of the depth.

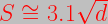
$S \cong 3.1 \sqrt{d}$

where d is the water’s depth (in meters).

but in all cases, it is much less than the speed of light. If an observer were to watch the crest of a wave as it moved along a seawall, or along any imaginary line that wasn’t along the wave’s direction of travel (directly away from a point source, or in the direction of the wind, or perpendicular to the wavefront, etc.), then the apparent speed would be greater than the calculated or expected speed, and as the angle of that reference line approached 90° to the direction of travel, the apparent speed would approach infinity.

To see the Note click here.To hide the Note click here.

$A = \frac{S}{\cos \theta}$

where A is the apparent speed, S is the expected speed, and θ is the angle between the reference line and the direction of travel.

which is well above the speed of light. As far as I know, this has no implications or gives no reason for hope for wannabe time travelers.

In Summary

So now you see my dilemma. To repeat the title plea, please help me understand. A crucial early step in solving any problem may be asking the right questions. Finding those should be as important, and in some cases may be as difficult as answering them. So let’s get started. Thank you for your help. If I do figure it out without your help, I’ll let you know.

The Walmart Donation-Matching Gimmick/Scam

The other day, I saw an advertisement/announcement on TV stating that for every dollar you contributed to certain causes, Walmart would give two dollars^Details. I was impressed for about thirty seconds.

History

Many companies have done matching-donation campaigns, whereby if you give a dollar, they will give a dollar. It can be effective in promoting more charity by the public in some communities. Or it could be a gimmick. It could just be an excuse to limit their donation. “We can afford to budget one million dollars, but if you can’t find matchers, I’ll just pocket the difference”. Usually they mention a cap or maximum donation that they are willing to make. That’s where the real deceit comes in.

Donation Limits

These limits sound reasonable for ensuring the donor company doesn’t get in over their heads by misjudging popular support and becoming committed to a donation they cannot afford. But these companies have already done their homework. For example, if the Green Cross (names in these examples have been changed just to keep you from thinking these principles have limited applicability) Christmas campaign raised ten million dollars last year and has grown as much as 20% year to year (with many years much less), an observer would have reason to be cynical if the donation-matching company has a donation limit of much less than about 15 million dollars.

Typically, the donor company will impose a limit of one or two million dollars. I’m sure their maximum donation will be much less than the cost their advertising company would charge for similar exposure. But when the donation-matching company imposes such a low limit, their actual final donation will always be equal to that limit. The donor company may not advertise reaching the limit, and the deception of the public will begin.

The ethical thing would have to just announce they were making a simple donation of one million dollars. But if you can’t fool suckers into coughing up more money (mistakenly thinking their donation is worth twice as much to the charity), where is the sport in that. At least this time it is for a good cause.

Walmart Doubles Down

Walmart’s new wrinkle takes this scam to the next level. But all it means is that the owners of Walmart will reach their company donation limit sooner, and will be able to laugh at the stupidity of even more Americans. That’s called arrogance.

Suppose that in this year’s campaign, the Green Cross is expecting ten million Americans to each donate a dollar (I’m just making the math simple). Under their old plan, Acme Widgets would make their generous four-million-dollar-donation by announcing they will match your donation dollar-for-dollar (for up to four million of their dollars). The first four million Americans bring in eight million dollars for the Green Cross (including Acme’s portion). If the remaining six million Americans donate expecting to see their money go further, they are being misled. They will bring in just six million dollars, and the Green Cross total for that year will be $14 million – a clear victory for the Green Cross (and Acme).

Under their new plan, Acme will now make their budgeted four-million-dollar-donation by announcing they will match your donation with, say, four dollars for each of yours (for up to one million of your dollars). Now Acme reaches their limit much faster. The first million Americans, with matching funds, help the Green Cross bring in five million dollars. The last nine million donors (up 50% from last year) will make a contribution that they mistakenly believe is being amplified. They will contribute only their own nine million dollars, bringing the Green Cross the same $14 million as last year. Acme will contribute the same amount. And the same amount will come from individual donors, 90% of whom were deceived.

Disclaimers

I am not suggesting here that donating to charity is bad; quite the contrary. I believe that helping others is a good idea. The Bible even supports that idea. But there are a few things one should consider:

Are you donating for the right reasons? Donations with strings attached aren’t really donations. You should not donate expecting to get anything in return (not even a reserved seat in the Hereafter). Your rewards should be internal.
Similarly, don’t assume, think, or claim the recipient of your donation will place a higher value on it than you do; that’s just fraud. I’ve recently heard complaints from members of relief organizations accepting donations for victims of Hurricane Harvey about people donating expired or opened food, worn-out clothes, etc. You are not fooling anybody.
On the other hand, are you giving within your means? Creating a hardship for one person to ease another – the saying for that is “Robbing Peter to pay Paul” (a Biblical reference, but not from the Bible), is not recommended. Even the airlines advise you to put the oxygen mask on yourself before tending to your children.
Finally, not all charities are created equal. And not all charities are the best stewards of your money. I suggest doing your homework;
After checking out a charity’s website, you can research potential donees at places like Charity Navigator.

What Next?

As time permits, I will be discussing some ways advertisers use math to deceive their customers. Stay tuned.

We Can Simplify Our Student Grading System

In the works, I have two different questions for you:

‘Do Medium-sized Egos Really Exist?’, and
‘Should Law Enforcement Officers Be Allowed To Use The “I was afraid for my life” Defense?’

Both of these require some preparation/research, but I hope to have them ready before too long. For now, I’ve chosen a lighter topic about a scheme that, because it’s not being implemented as designed, could well be simplified.

Some Background

When I started school, we got one grade, from A to F (I never learned why E was left out), to represent our mastery of the subject. Then, at some point, they introduced a separate grade for effort (from 1 to 3) and another for conduct (also A through F, also without the E). These were promoted as independent variables that could give more insight into the performance of one’s child. I soon had reason to question the independence of these variables.

What’s The Best Grade You Can Get

Conventional wisdom tells us that the highest grade one can get would now be an A1A. I’m not here to discuss the merits of bad behavior, so we will focus only on the first two symbols. To me, it was obvious that an A3 would be more desirable. Here’s why –

Suppose it’s a leap year and you are betting on track events at the Summer Olympics. In the first heat, the first place runner comes in with a time of, say, 4:00.00. At the end she is visibly spent (lying on the ground, breathing heavily, and sweating profusely). Her grade would clearly be an A1. In the next heat, the winner has the exact same time but isn’t even breathing hard. I would give her an A3. Keep in mind that it is not uncommon for runners in the early heats of big events to pace themselves to save some effort for later heats, if they can afford to.

Of course, both runners advance to the finals with the best times. Again, conventional wisdom gives the higher grade to the first runner. But tell the truth – which one are you betting your hard-earned money on in the finals?

My Experience

So you can see what grade I was trying for. But the truth is teachers don’t give A3 grades, even if you never turn in your homework. This isn’t a case of political correctness (whereby we fashion our remarks based on the possible objections of imaginary people with hyper thin skins or real fools priding themselves on how easily offended they can be). It is another common problem in the political arena whereby people refuse to let facts get in the way of their idea of the way things should work in their perfect (but grossly oversimplified) world. In their view, the very fact that you got an A proves that you were trying really hard because hard honest work is what made America great.

The problem is that once you link those previously independent variables (effort and results), you are really only working in a one-dimensional world. You don’t need two grades to adequately describe it.

Looking From The Other Side

But you may be saying to yourself “Silent, you are the anomaly! Only the very rare person who can find a task at which they can succeed without unbelievable effort would have the luxury of taking your position on this topic”. If you really think failure is the norm, then answer this:

Do you really think someone who, for whatever reason, didn’t meet the minimum requirements for success in this class, would prefer an F1 over an F3? From what I’ve observed, the opposite has usually been the case. If you give him an F1 you are saying “bless his little heart, he gave it his best shot but is just too stupid to make the grade”. That may even be true, but giving him an F3 gives him an alibi (or more accurately, reinforces the excuses he’s been giving even without your blessing) that he’s really very, very intelligent, but just didn’t put forth the effort.

I’m not saying to give all failures a “3” – you could just make the variable independent, open your eyes, and give the student whatever they truly earned.

Conclusion

There are actually two ways to cure this problem: we could start treating effort and results as the independent variable they are (which is probably too agonizing a task for most teachers). Or we could just stop giving the effort grade. I propose the latter. What do you think?

Not Quite Clear On The Concept (Part 1)

Earlier this month, the Catholic archdiocese of Newark, New Jersey, decreed that after four years of Catholic Youth Organization (CYO) basketball together, the St. John’s 5^th grade team (nine boys and two girls) would not be allowed to play the last two games of the season with girls on the team^A.

First, A Little Math

The maximum of any subset cannot be greater than the set maximum. This means that if the largest member of your weight-watching group is, say 400 pounds, then as people leave the group, that maximum will not get instantaneously larger. It could remain 400 pounds for a while, but will eventually get smaller as people continue to leave.

To see the Note click here.To hide the Note click here.

The average group weight, on the other hand, could go up or down depending on how people are selected for removal from the group, but averages aren’t generally used to measure greatness.

Similarly, in sports you cannot raise the level of competition by restricting participation. This means that you can’t say your team is the best in the universe if any member of the universe was barred from competing. Consequently, the only logical reason for restricting membership would be to protect the members from unfair competition. A team would only ban girls if they thought their boys weren’t ready for real competition.

Motives

As we all know, a group’s stated reasons for an action may differ from their real reasons. I suspect the archdiocese’s advertised reason for the decree is to protect girls from competition they can’t handle. But for that argument to have any credibility at all, at least two new conditions would have to be in effect:

There would actually have to be a girl’s team if you want anybody to believe that their interests are really your first priority.
You would protect a “weaker” group by banning the unfair competition from that group, not banning the allegedly weaker competition from the “stronger” or open group. The later option will rightly cause others to question your motives. “Who are you really protecting?”

The required game forfeitures would be further evidence of their true motive. A team should be required to forfeit a game only if they won using an unfair advantage. You would not make a boxer forfeit all the matches he won with one hand tied behind his back. Obviously, the other boys’ teams not only considered the girls a threat, but most likely the sole reason for the team’s success.

A Happy Ending

On hearing the decree, the St. John’s 5^th grade team immediately and unanimously decided to stick with their teammates and forfeit the season.

The girls, understandably, felt bad and offered to sacrifice themselves^A. St. John’s athletic director honorably rejected that offer. (In the body of that article, it suggests that the league director had already cancelled St. John’s season, making the athletic director’s gesture moot.)

A new Cardinal reversed the ban and allows St. John’s to play^A.

A Not-So-Happy Ending: Politics Trumps Logic

I just read about a different, but logically related case in Texas^A. A girl was taking testosterone to become a boy and wanted to compete with boys. New rules for the state of Texas required her to compete as a girl. She won their state wrestling championship. Not everybody was happy with her. I’ll leave the application of principle and subsequent comments to the reader.

Simple English: The Problem With The “If” Statement

You are probably very proud of your grasp of English (unless you live in South Florida, in which case you may not give a damn). And yet I have seen plenty of people whose lack of understanding about basic structures like the “If” statement

To see the Note click here.To hide the Note click here.

The next article in this series will discuss how misunderstandings about the conjunction “or” have caused so much trouble.

cause them to make terrible assumptions.

An Example

Suppose a young child is misbehaving to the point that the caregiving parent decrees “If you don’t knock that off, I’m going to paddle you” (This is an old example; I’m sure nobody would ever actually do that today 😉 ). As young children have been known to do, for whatever reason, the child continues with its behavior. The parent repeats the statement, with added emphasis. Nothing changes. The parent soon throws their hands up and says “wait until (your other parent) gets home”.

The parent’s first decree, like all “if” statements, had two parts; a condition and a consequence (joined by the conjunction “if”), with the understanding that if the condition is true, then the consequence will occur. It’s simple enough that even a young child can understand it. If the condition is met and the consequence is not accomplished, then the statement would be considered false. In short, the child knew that the parent was lying.

Now suppose the non-caregiving parent comes home, sees the objectionable behavior, makes a similar decree, and then the first parent points out that they had already made that decree to no avail. The child, for whatever reason, stops the objectionable behavior. To everybody’s surprise, the second parent paddles the child. Although the child and many of you listeners may think bad thoughts about this parent, one thing you can’t call him/her is a liar.

The Problem

As you can see here, the problem with the “if” statement is that is incomplete in the sense that it only addresses what happens when the condition is true, remaining completely silent to the possibility that the condition could be false. This allows most people to make the assumption that if the condition is false, the opposite of the consequence must occur. As the young child in our example learned, that assumption would be a mistake.

The Solution

Don’t make stupid assumptions. As your lawyer would tell you, get it in writing. In the above example, since the second parent didn’t make any promises about what would happen if the behavior did stop, s/he can’t be accused of lying. If this example bothers you, I’m sure the second parent told the child afterward that the paddling was for not obeying the first parent, in which case we would be unable to judge the truthfulness of their claim until after the right set of conditions are met following some later episode of misbehavior (guesstimating any change in likelihood of that future misbehavior based on recent events will be left as an exercise for the reader). To lawyers, mathematicians, and the like, the parent’s explanation doesn’t matter to this case and is unnecessary.

P.S.

Logicians have named operators (or functions) fulfilling all sixteen patterns of truthfulness or falsehood of expressions based on the truthfulness or falsity of two variables, such as the condition and consequence of the “if” statement described above. Engineers call the statement that yields the results you thought the “if” statement provided the “exclusive nor” function, “nor” being short for not or, meaning “giving the opposite results than the ‘or’ function”. Some refer to it as logical equality. In English, it would be represented by a sentence including the phrase “If, and only if”, such as “I will ground you for the rest of your life if, and only if, you do not stop screaming this very second”. If that type of statement had been the norm in this household, the non-caregiving parent, upon hearing the lack of results achieved by the other parent, was still free to add other (most likely “or”) clauses (to be discussed in a later article) to his/her decree. If you are now totally confused, please do not sign any document containing more than six words before consulting an attorney, or at least a mathematician. On second thought, in cases like this, I would stick with the lawyer.

How America’s Cup Committee Stole Victory From Kiwis

The America’s Cup, a yacht race between a single defender and a single challenger. It was first run in 1851 by the Royal Yacht Squadron in England for a race around the Isle of Wight, and is known as the oldest international sporting trophy^A. The referenced Wikipedia article provides an excellent history of the contest. It notes that the Americans held the cup continuously from the first race until 1983. That is the longest winning streak in the history of sport.

The cup was last contested in San Francisco in 2013, but this wasn’t your father’s America’s Cup. The vessels were now 72-foot catamarans (boats with two hulls) costing well over ten million dollars each. They have rigid “sails” and foils (short underwater wings) that lifted the entire hull out of the water. Their top speed was over 50 miles an hour, or about twice the wind speed. The whole race was held within sight of spectators ashore. And instead of taking all day, as was customary, races were finished in less than forty minutes to fit snugly between commercials in a one-hour television format.

The series also turned out to have one of the greatest comebacks in sports. Stu Woo of the Wall Street Journal did an outstanding job of explaining that competition^A. Stu failed to mention a minor change in the rules that bought the American team just enough time to complete the changes necessary to turn things around.

How it Happened

The 2013 America’s Cup was originally billed as a best-of-seventeen series.

To see the Note click here.To hide the Note click here.

October 7, 2018 Update: Notified of a broken link, I see all official mention of the best-of-seventeen aspect of the competition has been scrubbed. After looking through multiple websites, I did find it mentioned in the third-to-last paragraph of a third-party article, “The Miracle on San Francisco Bay” at www.slate.com.

A Best-of-T series (where T represents the total number of games to be played and is always an odd number) is widely used in sports championships. (But before September 2013 I had never heard of T being larger than seven.) For those of you who are not sports fans, that means that the two teams will compete exactly T times. (We will use seventeen fot T in the rest of our examples.) Then count up each teams wins to name the victor. One team can declare themselves the winner and send everybody home early if they can accumulate more of a lead than the other team can overcome in the remainder of the seventeen games. In a simple world, meaning a world without ties or penalty points, that would be nine wins in a best-of-seventeen series ((17 ÷ 2) rounded up).

Yacht racing, as the references in the first paragraph suggested, is not a simple world. Before the series started, the American team was penalized two points for cheating in an earlier round of competition. That meant their first two wins wouldn’t really count (except to keep points away from their competitor). And although the calendar continued to list seventeen races (with the caveat “if needed” as appropriate) well into the competition^D, all of the experts were still saying that it would take nine wins for the Kiwis to take home the trophy.

The truth is that after the seventeenth race had been sailed, if New Zealand had only eight wins, then the Americans would have won nine races (17 – 8 = 9), but would only have had seven points (9 – 2 = 7). That means that New Zealand would have taken home the trophy. That is what a best-of-seventeen series really means. And, as you can see near the bottom of the Final Scorecard at the end of Stu Woo’s Wall Street Journal article, that is exactly what happened.

But just before New Zealand earned their eighth victory in Race 11, the race committee declared that because the American team’s penalty shouldn’t affect New Zealand, they would still need nine wins to take home the trophy. Shortly thereafter Races 18 & 19 were added to the schedule. As we now know, thanks to a brilliant turn-around by the American sailors, New Zealand never got that ninth win. The American’s got that point in the nineteenth and final race. Competition doesn’t get any better than that.

But had the Kiwis been able to count up to seventeen or had their English been good enough to understand the meaning of “best of seventeen races”, the ending of this story would have been completely different.

The Art Of Communication

I like the definition of communication given by Wikipedia that talks about a process of reaching mutual understanding, where participants not only exchange information but also create and share meaning. This is a process that obviously takes more than one person.

That point was apparently lost on me as a child. At that time, there were quite a few of my fellow Southern Californians that spoke Spanish. And yet when it came time to choose a language to study in school, as required, and having a choice of Spanish, French, German, and possibly Italian and Japanese, I picked German because I wanted to be different. I studied the language for six years and like to think I was decent at it. But then again, there were no Germans (to speak of) around to contradict me. Many years have gone by and I can still count past twelve, but some might argue that I didn’t get the most bang for my educational buck. Now I am surrounded by people in Southern Florida, some who have been visiting for over fifty years, who still have trouble with English and don’t understand why everybody here doesn’t just speak Spanish.

But I am not the only one who has struggled with the concept.

The T-shirt

Several weeks ago I saw a gentleman walking down the street wearing a T-shirt with the following sign:

To see the Note click here.To hide the Note click here.

To translate, the first symbol is the square root of negative one, which technically doesn’t exist, but is more commonly represented by the small letter “i” as the basis for all imaginary numbers. The second expression, two to the third power (2x2x2), reduces to eight. The third, the capital Greek letter Sigma, is seldom seen alone because it represents the sum of the sequence that follows. The last symbol is the small Greek letter Pi, which has come to be known as the most famous irrational (meaning it never ends and never repeats) constant – representing, among other things, the ratio of a circle’s circumference to its diameter.

The common name of each of these symbols resembles an unrelated English word. So the expression can be pronounced “I ate some pie”. But what was the wearer really trying to say? Mathematically, the expression is meaningless. Its sole purpose seems to be to announce to a select audience what was on his lunch menu. Maybe the fewer the people who knew that he went off of his diet, the better. This may be an attempt at a confession without guilt. While that seemed harmless enough, it reminded me of another obscure message I had seen before.

The Jeep

While driving down the road ages ago, a Jeep passed me that had the following array of small international maritime signal flags displayed on its back window:

International maritime signal flags spelling F.U.C.K. Y.O.U.

Each of these flags, which are used by navies and merchant marines around the world, has a name taken from the phonetic alphabet. Along the top row is Foxtrot, Uniform, Charlie, and Kilo. On the second row sits Yankee, Oscar, and Uniform again. Individually, each flag has a meaning. For example, Oscar means someone has fallen overboard. In small, seemingly random groups they could represent short code words. But sometimes they just represent the letter at the beginning of their name.

To see the Note click here.To hide the Note click here.

If you want to try to impress your friends at parties, I just found out the term for that (the naming of letters in an alphabet so that the name begins with that letter) is called acrophony^D.

Although I thought the Jeep owner’s words were clear enough, I wasn’t quite sure about his/her message. Was this message for general audiences? in that case the owner is an ineffective coward, but was probably getting a chuckle imagining himself (or herself) smarter than every other person on the road and able to insult them with impunity. Or did s/he have some problem specifically with sailors? Maybe that’s what s/he wanted to be when they grew up, but either got seasick too easily or didn’t work well with others in confined spaces, and so was taking their frustrations at his/her own inadequacies out on the very group they wanted to be part of. Who knows? I followed the car into a parking lot and after s/he went inside I carved the phrase “Roger, out!” into their back tire. The tire went flat when I applied the punctuation.

To see the Note click here.To hide the Note click here.

In radio communications, “Roger” simply means the message was received and understood^D. It does not mean agreement. “Out”, as I’ve mentioned before^A, means “and this conversation is finished.”.

To see the Note click here.To hide the Note click here.

Although based on a true story, the ending of the last paragraph was changed to better illustrate the range of possible consequences one should expect for one’s actions. The truth is that when s/he turned into the parking lot, I just kept driving. I decided they couldn’t possibly have been talking to me. (Luke 23:34^D comes to mind.)